19th European Intensive Course

on Complex Analysis, its Generalizations and Applications

TOPICS

The 19th European Intensive Course, March 17-28, 2014, to be held at the University of Aveiro, will have a total of 18 hours of lecture time, at postgraduate level, introducing topics of high current interest. This year the Intensive Course will be centered around the following topics:

Inverse Problems, Harmonic Analysis, and Representation Theory

During the course there will be extended coffee breaks in a relaxed environment where lecturers and participants are encouraged to share ideas in a more informal way. Successfully participating students will get a certificate.

Intertwining the two weeks of the course, we announce the 16th Annual Workshop on Applications and Generalizations of Complex Analysis, to be held on March 21-22, 2014.

POSTER of the 19th EIC

LECTURERS - Tentative schedule, titles & abstracts

Lecture Room - Sala Sousa Pinto (room equipped with beamer and white board)

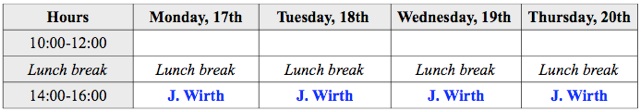
1st week, March 17-20

Jens Wirth, Universität Stuttgart, Germany

A Rough Guide to Non-Commutative Phase Space

We present a rough guide to operator theory on compact Lie groups and associated global symbolic calculi. The course will be organised as follows:

(1) Basics:

1. • Peter--Weyl theorem and (noncommutative) Fourier transform on compact Lie groups.
   

2. • Examples: Tori and S^3.
   

3. • Function spaces and their Fourier characterisation.
   

4. • Schwartz kernels of operators, right-convolution kernels.
   

(2) Differential operators and difference operators

1. • (Left-) Invariant differential operators and their symbols.
   

2. • What are difference operators acting on symbols?
   

3. • Calculus rules for difference operators.
   

4. • Taylor expansions and admissibility.
   

(3) Functional calculus of left-invariant operators

1. • Analytic functional calculus for matrices. 
   

2. • Key lemmata.
   

3. • Bounded symbols and operators, unbounded operators.
   

(4) Symbol classes and pseudo-differential operators

1. • Symbol classes and asymptotic expansions.
   

2. • Basic calculus statements.
   

3. • Ellipticity.
   

4. • Boundedness.
   

5. • Functional calculus.
   

6. • Operator classes characterised by symbols.
   

It will be assumed that participants have basic knowledge in contemporary analysis, in particular concerning distribution theory, trigonometric series and the analysis of differential operators.

*****

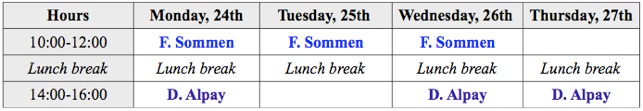
2nd week, March 24-28

Frank Sommen, Ghent University, Belgium

Introduction to Clifford Analysis 

Lectures notes: pdf1  pdf2

Daniel Alpay, Ben-Gurion University of the Negev, Israel   

Infinite dimensional analysis, non commutative stochastic distributions and applications

Lectures notes: pdf

Lecture 1:  Countably normed spaces, their duals and Gelfand triples

Lecture 2:  Positive definite functions, Bochner and Bochner-Minlos theorem. Hida's white noise space and Kondratiev's spaces of stochastic distributions

Lecture 3: Stationary increments stochastic processes. Linear stochastic systems.

Lecture 4: Fock spaces and non commutative stochastic distributions

Lecture 5: The free setting. Free (non commutative) stochastic processes.

Lecture 6: A new class of topological algebras

THE ORGANIZERS       

Uwe Kaehler                   
Paula Cerejeiras

Departamento de Matemática, Universidade de Aveiro                   
Campus de Santiago
P-3810-193 Aveiro 
Portugal 

fax: +351-234370066                                    tel: +351-234370359                                  e-mails: ukaehler@ua.pt   pceres@ua.pt

These events are supported by Portuguese funds through the CIDMA - Center for Research and Development in Mathematics and Applications, and the Portuguese Foundation for Science and Technology (“FCT–Fundação para a Ciência e a Tecnologia”), within project PEst-OE/MAT/UI4106/2014.